[HOW TO]Calculate the Volume Occupied by Torispherical Dish

Got a query asking How to Calculate the volume occupied by torispherical dish of a batch reactor,
this query will definitely have a solution, but usually many engineers will keep following some of the thumb rules those are applicable here, and some of the others will apply whatever thumb they remember, so its better to use thumb rule practically, but before that its very recommend to know the reason behind deriving the thumb.




So before going into calculation directly, first of all we should know some basic concepts involved in the design of torispherical dish [doom/disc].,

** Crown Radius: A torispherical dish is the surface obtained from the intersection of a spherical cap with a tangent torus, as Shown in above pic. The radius of the sphere R is called the "crown radius,"

** Knuckle Radius: The Radius of the circle's those are formed at the transition phase from spherical surface to flat surface is called Knuckle Radius, Usually the sphere's / circle's those were formed at those transition phases were called Torus, and in plural form mentioned as Torii, so having torii is the reason behind deriving the name as torispherical dishes, And 

generally the knucle radius of a torispherical dish will be 0.6 times the diameter of the vessel.

Usually these torispherical dishes were designed for the sake of pressure vessels and storage vessels, to avoid the breathing losses in such vessels these dishes were designed.

You May Also Like:

Types of Agitators and their Significance  

What does Tonnes of Refrigeration means? 

How to Select a Condenser?

** Critical Radius: The Radius where the transition occurs from Spherical to Torus is called as Critical Radius.,



   
So, if you are clear with the above basic definitions we can enter into calculation part,

Let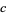  be the distance from the center of the torus to the center of the torus tube, let 'c' be the radius of the torus tube, and let 'h' be the height from the base of the dome to the top. Then the radius of the base is given by a + c < R. In addition, by elementary geometry, a torispherical dome satisfies 

The transition from sphere to torus occurs at the critical radius 

So from the basic Dome equation from 3d Co-ordinate geometry, we are having two equations based on different cases

Where,

The Torispherical Dome has Volume

So, these are the theoretical Myth that our thumb is basing , and now i'm gonna tell you the thumb for calculating the Torispherical end volume,




Volume occupied by torispherical dish, V= (Pi/24)* Di*Di*Di.,

And some people want to have some clarity even through Thumb, so for them practice this,

Volume, V= [0.0847*Di*Di*Di]+ [ (pi*Di*Di*S.F)/4].

Thats it......Cheers,




Comments are appreciated......!!!!!

Some of the Readers wont have much time to read and digest all the above theory and may need instant results, for them i have developed an excel sheet, use that sheet for calculation,

DOWNLOAD EXCEL SHEET HERE

You May Also Like:

How to Calculate Energy of steam?  

What does Tonnes of Refrigeration means? 

How to Select a Condenser? 

Determine the Power Required for an Operation?  

How to Calculate required Vapour Column Size?

About The Author

---

Hi! I am Ajay Kumar Kalva, Currently serving as the CEO of this site, a tech geek by passion, and a chemical process engineer by profession, i'm interested in writing articles regarding technology, hacking and pharma technology.
Follow Me on Twitter AjaySpectator & Computer Innovations